


Next: Bibliography
Up: Collectivity of the low-lying
Previous: Onset of collective low-energy
In the present work, we have applied the relativistic mean
field model to describe ground state and
collective properties of E1 excitations in atomic nuclei.
The framework of relativistic random phase approximation
has been used to investigate possible soft isovector dipole modes
(pygmy resonances) through the periodic table. It has been shown that
the RRPA isovector dipole strength consists of two parts: the
well known giant dipole resonance, and separated low energy mode.
In general, low lying dipole strength increase relatively more
when compared with the GDR strength, as the number of neutrons
increase.
RRPA approach have been applied to analyze the
collectivity of dipole excitations in the region of the low-lying dipole
strength. The collective nuclear properties of the low-energy states which
exhausts a few percent of energy weighted sum rule, have been
investigated through the periodic table. In particular we have analyzed the
low-lying dipole strength by separating the contributions from RRPA
amplitudes of each particle-hole configuration to the RRPA eigenvalues.
The relationship between the
neutron particle-hole excitations in the low-energy region and the
existence of collective pygmy modes have been investigated. Calculations
are performed with the standard nonlinear parameterization NL3 without
additional adjustments,
here resulting with the isovector giant resonances which obey the
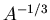 law. In RRPA analysis, properties of the low-lying modes
have been systematically compared with the corresponding giant
resonances, to display the difference between the two modes.
In the case of light neutron rich
nuclei, in addition to the giant dipole resonance, we observe the onset
of low-lying dipole strength
corresponding mainly to the strong contribution of the single particle-hole
excitations, without evidence of collectivity in these modes.
As the mass number increase, more particle-hole configurations play a
significant role in the low-energy states. The degree of collectivity
increases with the mass number, and evidence of collective
pygmy resonances is identified in the medium-heavy nuclei.
Transition densities of low-energy peaks are characterized
with a neutron dominated tail beyond the nuclear surface, with proton
and neutron transition densities oscillating in phase in the internal
region of nucleus. In comparison, the proton and neutron transition
densities of the GDR mode have opposite signs, and both have similar
contributions. The study of transition currents have been applied to
investigate the collective low-lying dipole modes in medium nuclei.
The corresponding velocity fields have disclosed the dynamical properties
of soft dipole resonances. Accordingly, neutron excess oscillates
against the symmetric proton-neutron core occupying the same orbitals,
with main out of phase behavior in the region close to the surface region.
Collective description with velocity fields makes possibillity of comparing
the soft modes with simple hydrodynamical models describing similar
dynamics of low-lying modes.
But in these models oscillations of excess neutrons against the rest
of nucleons are obtained as a result of such initial description of the
system. In contrast, we describe dynamics of pygmy resonances
in microscopic self-consistent approach, without special adjustments
to a particular low-energy mode. Resulting properties of RRPA velocity
fields nicely fits into the hydrodynamical assumption of two-fluid model.
In the heavy nuclei, the existence of the collective low-energy
pygmy mode is well established, characterized with many comparable neutron
particle-hole contributions dominating against the response of proton p-h
configurations.
law. In RRPA analysis, properties of the low-lying modes
have been systematically compared with the corresponding giant
resonances, to display the difference between the two modes.
In the case of light neutron rich
nuclei, in addition to the giant dipole resonance, we observe the onset
of low-lying dipole strength
corresponding mainly to the strong contribution of the single particle-hole
excitations, without evidence of collectivity in these modes.
As the mass number increase, more particle-hole configurations play a
significant role in the low-energy states. The degree of collectivity
increases with the mass number, and evidence of collective
pygmy resonances is identified in the medium-heavy nuclei.
Transition densities of low-energy peaks are characterized
with a neutron dominated tail beyond the nuclear surface, with proton
and neutron transition densities oscillating in phase in the internal
region of nucleus. In comparison, the proton and neutron transition
densities of the GDR mode have opposite signs, and both have similar
contributions. The study of transition currents have been applied to
investigate the collective low-lying dipole modes in medium nuclei.
The corresponding velocity fields have disclosed the dynamical properties
of soft dipole resonances. Accordingly, neutron excess oscillates
against the symmetric proton-neutron core occupying the same orbitals,
with main out of phase behavior in the region close to the surface region.
Collective description with velocity fields makes possibillity of comparing
the soft modes with simple hydrodynamical models describing similar
dynamics of low-lying modes.
But in these models oscillations of excess neutrons against the rest
of nucleons are obtained as a result of such initial description of the
system. In contrast, we describe dynamics of pygmy resonances
in microscopic self-consistent approach, without special adjustments
to a particular low-energy mode. Resulting properties of RRPA velocity
fields nicely fits into the hydrodynamical assumption of two-fluid model.
In the heavy nuclei, the existence of the collective low-energy
pygmy mode is well established, characterized with many comparable neutron
particle-hole contributions dominating against the response of proton p-h
configurations.
Acknowledgments
This work has been supported in part by the
Bundesministerium für Bildung und Forschung under
contract 06 TM 979, by the Deutsche Forschungsgemeinschaft,
and by the Gesellschaft für Schwerionenforschung (GSI)
Darmstadt.



Next: Bibliography
Up: Collectivity of the low-lying
Previous: Onset of collective low-energy
Nils Paar, 2001.

